Observation #2 Reflection

For this observation, My CT allowed me to choose what part of the lesson in which i would like to teach, the notes section or the activity section. I choose to do the activity. I created an activity based off a book activity, but changed it to apply to the culture of the students. The activity was of an E-statement of a bank account and the activity of one month. When I had the students do the first calculation, instead of doing the long way of subtraction numbers, they grab their calculators right away. Right away I need this activity was not going to be hard for students if they understood how the table was set up. If I teach this activity again, I would allow blanks in the statement in which they are allowed to put what they send their money on or how much money is given to them. This will allow a vary of answers and for the students who understand will (hopefully) choose some interesting numbers. I would also add a couple more deep thinking questions. If the students understand the process, they can get a deeper connection to the real-life application.

When Jon came in, I asked him to write down the questions that I asked my students during the activity. When I looked back at the questions I asked, I was trying to understand how each student was thinking. As a first year teacher, I still am unsure of how the students think. Asking the simple question of "how did you get that answer?" or "can you explain yourself?". I hope I keep using these questions more and become aware of my students thinking.

Cheat Sheets in the Classroom...Yes or No?

The above question popped into my mind when my CT was passing out a haft sheet of pink paper that had "short cuts" for transformations. She informed the students that they will be able to use this paper for the test, but she know how to do transformations in general for the MEAP test. I thought in my head, "you are allowing the students to use this cheat sheet when you know they will not be given this information on a standardized test". As I walked around the classroom when the students were working on their classwork, I noticed that the cheat sheet did help the students to get t the correct answer, but do they truly understand the reasoning behind the transformation. Or are they showing the skill of looking onto the cheat sheet for the answer, the "plug-and-chug" method.

I looked back on my education and when I was able to use a cheat sheet. I was given a "formula sheet" for Algebra 2/Trig and Calculus in High School. Thinking back, I realize I did not fully understand the material and when i got stuck on a problem in math class I looked to that sheet for the answer in one of the formulas. So did i really understand the big ideas...i would say no i did not understand.

So are we helping or hurting our students by allowing them to have a cheat sheet for a test? Do we get cheat sheets in life? But at the same time, we do get a cheat sheet in everyday life...it is called the internet. We are able to look up any different things at the touch of a button. So does it matter if we allow students to use a cheat sheet?

Different Ways to Call on Students in the Classroom

In my class, we have a lot of examples we do together as a whole class. As I have noticed through the first couple of weeks of school the same few students will always have their hand raised up high to answer the questions. Even the "easy" questions are only answered by those students. I was surfing the internet and found a couple of different ways to call on students in the classroom.



1) Popsicle sticks: write the students names onto Popsicle sticks and pull out a name when you need a different student to answer the question.

2) "Calling on a different student" : the teacher would call on an arbitrary student and that student will be allowed to call onto the next students to answer the question. Once the students has been called on, they can not be called on again until everyone in the class has had a turn to answer a question.

3) "Phone a friend": This allows the students that you cold called who do not know the answer to the question to phone a friend. This allows the students to help each other out and do not feel pressure if they do not know the answer.

4) "Think, Pair, Share": The students will be give a couple of seconds to think of an answer to the question. Then they will look to their partner and share what they thought the answer was. Then the teacher can call onto one of the students in a group to share the answer they think is correct to the whole class.

I would love to figure out what technique works for my future students. If you have different ways to call on students in the classroom, please comment below. I would love to hear your way :)

Working TOGETHER in Math!

Students think that they are going to walk into "Math World" and enter by themselves and work on problems all alone and they will be able to know how to do each problem. My teacher is trying to break that idea of working alone in Math class. How she does breaks that idea is by forming "homework Groups".

HOMEWORK GROUPS
The students arrive to the classroom with just an answer key on the board with no work show for the problem. They check their homework and compare the answers to the answer key. When the bell rings for the class to start, Mrs. Ondrusek will allow the students to talk about their homework within a designated "homework group". This allows the students to ask their peers how to do a problem that they did not understand themselves. After about five minutes, Mrs. Ondrusek will allow a full class discussion about questions that no one in the group was able to answer correctly. After the class discussion, Mrs. Ondrusek will hand out a haft sheet of colored paper in which there are two simple questions the "homework group" has to answer:
1. We feel confident with...
2. We are still confused about...
This piece of paper will be turned in along with the homework. Mrs. Ondrusek and I read each of the groups haft papers and write little notes that will help them understand a little better. This allow us to look at what we need to go over again if the whole class does not understand something.

I think this is a great tool to be used in the classroom. We have not had an form of assessment yet to compare to the other way Mrs. Ondrusek has done in the past. We shall see in a couple weeks if she will continue this process of correcting homework.

My CT's classroom

The above image is a simple diagram of my teacher's classroom set up. Her largest class size is 34 students, but in her classroom she has 36 desk because she knows the finalized students schedules will not happen until the end of the week. She has the extra desks thinking she might get a couple of new students in the classroom. She would really want to put the students into their "homework groups" because then it would be easier for the students to work together not only on homework, but on the problems the book called "do it yourself" (she calls "work with a partner"). When she gets her classes finalized, she will group the student's desk (if she has room). She is also concerned that not all students will be able to see the front of the classroom. She does not want the students back to her, so that will create a challenge with how many students she has in her classes. She also has some restriction in the classroom, a LARGE air-conditioning unit and her teacher workstation with an Elmo and computer for the smart board. she does not want the students behind these objects in a way they will not be able to see what is going on in the front of the classroom.

Has technology really changed?

Society is telling the people that technology will influence our children's behavior and will miss social ques from peers because of how much cellphones and computers have taken their eyes from to world around them to a screen right in front of them. I say to you, now look at the two pictures to the left. One was taken on a train years ago and the other taken also on a train in the present day. In both pictures technology is taken the eye sight  of the people in the picture. In the old black and white picture it is the news paper, while in the present picture it is cellphones. So what is this difference between technology years ago compare to technology today? My spouse, Josh, once said "cellphones are a newer way to mind your own business". I truly believe that technology has not change the way our society functions, but technology has become more prevent in society. 

Black Jack in Math class...NO WAY!

When students hear "probability" or "statistics" in a math class, most student will protest and say they cant do that type of math. My approach to a probability lesson is not let them know what they are learning, but to have them learn and play a card game, Black Jack!

If you do not know the full rules of Black Jack, you are able to find them here.

The following is a lesson plan outline that I created. I have taught is in a college level classroom, but not in a high school classroom. I believe the lesson might have to be changed according to your students.

Review: factions/decimal/percent relation 

-Write on board different fractions

- have students draw a picture, write the percentage, and write the decimal form of each fraction

- allow students to mark what answers they think are wrong and what answers they think are correct

Hand out the following table and allow the students to find answers in groups (you might have to reword some the chance column to meet the need of your students):

In the table below, assume that you are the only player and only a single deck of cards is being used such that we do not reshuffle the cards into the deck.

Chance of:	number:
a red queen in either first two cards
two aces in either first two cards
a 21 in the first two cards
a 21 after the first card that is drawn is an 8
two “face” cards
two cards valued 2-9
two 10’s

Let the fun begin: Black Jack

- break the classroom up into smaller groups

- play a couple of "open hands" (all cards facing up) 

- have each student figure out the probability of busting if even another card base on the cards played at the table 

I created the following table for my students to use to keep track of their numbers: 

Total [of your two cards]	Probability of busting	What Happened

The college students really enjoyed this lesson. I hope I am able to use it in the classroom or even a smaller version with maybe only five cards for younger students. 

Co-teaching...What is That?!?!

The general definition of co-teaching involves two equally-qualified individuals who may or may not have the same area of expertise jointly delivering instruction to a group of students Co-teaching is something as upcoming teachers need to learn how to do. Co-teaching is a strategy that is in many classroom, weather it is with an special education teacher or another teacher that knows the subject. This strategy is to benefit the students as well as the head teacher.

There are five co-teaching approaches according to Jane M. Sileo are

1. One Teach, One Support

2. Team Teaching

3. Parallel Teaching

4. Station Teaching

5. Alternate Teaching  

Each of these approaches can but used throughout a unit in a classroom that host co-teachers. Picking the best approach for your classroom is the key in your students learning. We will break down each one and what each one entails.

One Teach, One Support

This approach entail that the "lead" teacher is teaching the whole class all at once. The other teacher will support the "lead" teacher by gathering observational data for the teacher or circulating the classroom providing assistance to  the students that need help.

Team Teaching

In this approach the teachers are teaching the material at the same time in the front of the classroom together. Another name for this approach is "tag team" or "one brain in two bodies". This could be in the form of a debate, modeling information, or role playing. The teachers have to prepare for the class together and make sure they know where the other teacher is and where they want the lesson to end.

Parallel Teaching

The class will be split into two groups and each teacher will teach the smaller group the same material. The students could be seeing the same material but in different ways, depending on the needs of each student.

Station Teaching

students are divided into three or four different smaller groups in which they rotate to different station in the classroom. At each station, there is different material or a different way to view the same material or concepts. The two teachers will be walking around the classroom helping students individually or as a group.

Alternative teaching

One teacher is teaching a larger group of the students, while the other teacher is telling a small group of students. This small group of students might just need some more re-teaching, pre-teaching, or enrichment. The key to this approach is that the larger group is not learning any new concepts/material, so the smaller group will not fall behind.

Each of these approaches have pros and cons, but you and your co-teacher will have to decide what one will work best with your group of students and your classroom.

In out project in MTH 329, we have to use one of the following approaches to teach our lesson. My group did a combination of team teach and stations teaching. I will let you know how the lesson goes with the co-teaching. 

UPDATE:
My group started off with a simple review activity  in which the students wrote on the board the answers to what a fraction looks like in decimal, picture, and percentage form. As the students finished, they looked each others work and wrote a check mark on the board next to the ones they believe were wrong. My co-teachers and I team taught the next section with asking each student to explain their check mark and why they think it is wrong. We then transitioned into the students get into groups and filling out a table that had the chances of different outcomes of a card game. Here, my co-teachers and I walked around the class looking over each students shoulder and stopping to help each student if they needed help. We then came back other and wrote down the answer and discussed the material and how each student came up with that answer (team teaching). The final activity we used station teaching. In each group, we knew what main point we had to hit with each group and how we would hit the points. With the station teaching we were able to develop more one-on-one interactions with the students and see if the students full understood the concept we were getting at with the lesson. 

 Sileo, Jane M. "Co-teaching: Getting to Know Your Partner." TEACHING Exceptional Children 43 (2011): 32-38. Web.

Math Fun Facts!

           As the students walk into a math classroom, the students have one thing on their mind, " math is so boring! Why do we have to learn it?" In Professor Su's  lecture, "My Favorite Math Facts", he talks about how starting off a class period with one math fun fact can change a students perspective about math. These math facts show the students that there is more to math than just numbers on a paper and calculus equations that need to be memorized.  I enjoyed the lecture that was given by Professor Su to the Grand Valley student in which he shared his favorite math facts that he has shown his students throughout his years of teaching. It was very interesting to see some of the facts that he presented, I have already seen throughout my years of being a math student. I wrote a proof about the nine point circle and how the points correlates to the midpoints, the feet of the altitude, and the orthocenter.  Professor Su also presented the different ways that the Pythagorean Theorem can be proven. In one of my classes I had to write a formal proof solving the Pythagorean Theorem in the way that made the best sense to me. Also with the Pythagorean Theorem, we discussed in my class how it is represented in neutral and spherical geometry. The last fun fact that I knew was the friendship theorem. I discussed this in two of my classes on different occasions and how it can be proven by using simple math.

            Professor Su also presented fun facts that I did not know to the student body that were very interesting. My favorite one that he showed was the "magic trick" of the black and red pair of cards. He uses this trick to show people his magical side but in reality it is just some simple math and arrangement of the deck of cards.

The Red and Black Card Trick Revealed

material

1) 52 deck of cards

2) one mathematician  

step-up: arrange the deck of cards every other color (black,red,.....black,red,black,red)

let the trick beginning

1) hand the deck of cards to a student already split in haft (important note: make sure when you split the deck the colors on the bottom are two different colors)

2) have the student shuffle the cards only one time by the bridge shuffle and give the deck of cards back to you

3) put the deck of cards behind your back and "pretend" to fish around for two different cards

4) Pick the first two cards off the top of the deck! If the trick was done correctly the two cards will always be a pair of black and red cards!

LET THE STUDENTS BE AMAZED!  

These simple math fun facts can help  students become interested in the world of numbers. One fun fact can show the students there is a reason in learning math and it is not so boring after all!

For more interesting math fun facts, click here. 

Will You Be Out Smarted By a Star??

I had three students  try to solve the following question:

In the star shown here the sum of the four numbers in any "line" is the same for each of the five "lines". The five missing numbers are 9,10,11,12,13. Which number is represented by R?

Student #1

I had the student read the question and tell me what he/she thinks the question is asking for them to do. The student answered, "each line has to add up to the same number".  The first line that the student looked at was  4-N-R-3 because it was easy for him/her to read from left to right. The student tried all combination of the number 9 with all the other combinations.  Then the student looked at line 1-N-H-7. He/she knew that N was a common number between the two lines so they had to be the same. The student had that number as 9 and then did all the combinations. The student realized that those combinations did not work so they worked with different combinations of all the numbers The student figured out two combinations of the two lines  that equal 30. The student then told me that he/she was going to find the letters that were in common in each line and have the line equal to 30. When the student added up the letters, he/she wrote them vertical each time and kept rewriting the numbers he/she already knew-1,3,4,5,7. The students told me that is was their way of thinking and adding the numbers up this way was easier for him/her to understand.

Student #2

I had the student read the question and tell me what he/she thought the question was asking for them to solve. The student though the question asked to "find the numbers that represent the letters".  This students looked at the point of the star and wrote the difference between the points of the star. He/she thought the difference might help find the missing numbers in the middle of the star. then the student added the points of the star together and found those numbers thinking that might help with finding the missing numbers.  The student realized that did not help him/her with the problem so he/she moved on to multiplying the point number by itself. The student found that 3 times 3 equals nine which is one of the number that could be in the middle. He/she thought that nine could be letters N and R because it lies on the line with three in it. The student then looked at the letter I and show that it was in the middle of 3 and 5, 3-I-5. Here the students stopped and i asked if they were confused. He/she said "yes", so we reread the question again. This time the student found that he/she was missing the part about the line in the question. So I asked what were the lines of the star. He/she answered 4-H-C-5 and 4-N-R-3. Then the students added the number 4,5and 3 together thinking this might help.  He/she was getting frustrated again, so we reread the question again and drew a new picture of the star. We broke down the star into the different lines and then the student realized that the lines all had to equal the same sum. Here the student was confused about how four different numbers could add up to the same number. I showed him/her a simple example of 1+2+3+4=10 and 5+2+2+1=10. Each sequence is different but they add up to the same number. I then asked the student what would he/she do now that h/she has this new information. The student told me that he/she would find the missing letters in the lines that add up to the same sum.

Student #3

I asked the student to read the question and tell me what the question is asking the student to solve. As the student was reading the question he/she circled 9,10,11,12,13 and the letters because "they go together, the numbers wills will replace the letters". The student then answered my question that the problem is having him/her solve for the missing letter with the numbers given in the lines of the star. The student recognized that there was five lines in the star and each one had four numbers. The student added the two numbers that he/she knew and wrote them under the star. Then he/she put these numbers in order from smallest to largest because he/she was able to see that the numbers were only one away from each other. The student then added one random  number from the list in the problem-9,10,11,12,13- to the numbers from the points being added together. He/she realized that only three of the lines add to the same sum, but then found the mistake that each line has four numbers not just three. The student then looked at what lines intersect each other at what letter. He/she wrote down the added point numbers and then put two plus sign to remind him/herself that there are two numbers/letters that have to be added together. The student wrote the letters that corresponded to the sum of the points to the left. The student then picked two random numbers to put in for I and R and found right away that those numbers would not work because both large number (point of star sum and given number) are paired together. The student then pick random number from the given list and found that these numbers wouldn't work because the intersection line needs an eight to make the lines equal and eight is not one of the numbers that was given. Then he/she picked another two numbers and found four out of the five lines equal each other, but he/she made a mistake after he/she checked the work. He/she used a given number twice. The student was so close, but he/he felt proud that she almost had the answer.

Speed Dating in Math Class

I was sitting in my observation classroom of Trevor Kuzee 7th grade math class. He told his students if they did everything today, they could do a game of speeding dating at the end of the day to review for the up coming quiz. All of the 7th grades became very excited for speeding dating, while i was thinking in my head: "Why in the world is he doing speed dating in his 7th grade classroom? What does this have to do with math?"

As the students finished their final practice quizzes on the computer, Trevor started passing out the papers for speed dating and of course he hands one to me. The sheet is not a questionnaire to fill out but 20 different math problems about what they went over in class for the day. He told his students that I would be included in the speed dating round and who is able to explain the game. 

Here is how the game goes: 

Speed Dating

Material: 

1. timer 

2. worksheet of math problems able to do within a set time 

3. pencils 

How To Play: 

1) As a class figure out what side of the table will be moving while the other side sits in place

2) Tell the students how long they have to work on the problem (ours was one minute)*

3) tell the students the number to work on (Trevor jumper around the page so they were not going in order) 

4) the students will work on the problem, and if they need help they can ask their "date" for help

5) when the time is up: call on a student to give the answer to the question they worked on 

6) change seats!

7) repeat 

*for harder problems allow more time for the students

Students thinking 

This game was a great way to see the student's thinking in action. I noticed some students did the whole worksheet in a couple of rounds and then just went desk to desk helping their "dates" with the problems if they needed it. I also noticed some students struggled with simple addition and subtraction of negative numbers and they used algebra titles on the side of the paper to help their thinking processes. I also helped a couple of students who though they had the correct answer but then told them to rethink the problem and they found their mistakes. This game also allows immediate feedback on the students work. They know whether they have the answer right or wrong because they have a partner who can confirm the answer and the also have a student telling the whole class what the answer should be. 

This is a great review game to use for students of any age for any type of Math class! Thank you Trevor for using this game the day I came in and observed! 

Fractions?!?!?!?!

Most middle school students cringe at the word "fractions". The do not fully understand the world of fractions and what it has to offer the mathematical community. The operations of fractions are hard to understand and the students just have to remember the rules of fractions.

We do not want the students repeat the rules, but apply the rules to real fractions. No middle school students like to sit at their desk all day and use a pencil and paper to solve equation after equation of fractions applying the rules, but to get up and move around. 

As I was suffering the internet to come up with some ideas for games that could use the operations of fractions, I came across this blog post from a teacher who applied a great game for her students to review fraction operations. Fractionopoly

This game could be applied at the end of a lesson in which the students know the operations. This is a great tool in which the students can work at their own pace, but also need to remember the rules to solve the equations quickly. 

You can also apply this game to earlier/later lessons in the year with addition, subtraction, multiplication of integer and decimals. 

I was thinking of a game that had to do with fractions with a simple 52 card deck. 

Fractional War

object of the game: the students will be able to recognize what fractions (mixed and non-mixed) is the smallest. 

material:

1. a deck of cards for each pair of students 

How to play: 

1. Each player will turn over two cards. 

       The first card will be the numerator. 

        The second card will be the denominator.

2. The player with the smallest simple fraction wins that hand. 

3. The player who collects all the cards wins! 

Notes: 

1. The fractions will have to be reduced to the simplest fraction. 

Example: If student #1 has 3/9 and student #2 has 6/12. Then student #1 wins because 3/9 is reduce-able to 1/3 which is smaller than 1/2. 

2. If there is a tie with the fractions: have the students draw another two cards. Whoever wins between the new two cards wins all the cards in that hand. 

Modifications:

A teacher could create new cards with all the operation signs on them. The students would first have to flip one of those up before they flip up their two cards. What ever the operation is, the students would have to do that operation with the two fractions that they created. Whoever comes up with an answer has to hit the table and say the answer. If the answer is correct, they win that hand! 

How do you know a game is good or bad for the concept you would like to teach?
I believe a math game should be able to connects many of the common core standards all into a single game. Also, a game can be built upon to new ideas and concepts. A game will have the students discover different ideas about one concept and then they are able to remember how they came up with ideas because they had a fun and interactive way of discovering the ideas. I believe good games should be open ended. What do I mean about "open ended"? The students are not looking for one answer and check to see if that is the answer the teacher got, but they are able to get different answers by going through the game in many directions. All games are fun to students even if you are a senior in high school. The students will remember more if they discovered it on their own in a fun away. 

Human Number Line Game

The definition of a number line according to Webster dictionary is a line of infinite extent whose points correspond to the real numbers according to their distance in a positive or negative direction from a point arbitrarily taken as zero. 

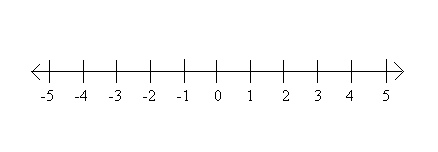
What does that word really mean to students? The number line is on a piece of paper that they can use if they understand it. I know when I was a students in middle school, I did not understand the number line fully until I played the game "Human Number Line" in my college class. 

Human Number Line Game

First, you will create a number line on the floor of the classroom or gym. The number line can be as long or as short as you wish and be created with tape or even string. The number line should include negative and positive number along with a zero. The numbers should be the same difference apart and close enough the students are able to take a step and be able to land on the next integers.  Below is a picture from a blog that has a similar game. 

The game will teach students how to add and subtract positive and negative integers. You will first allow the students to play with the number line by telling them these simple rules: 

1. start neutral (feet facing a wall) at the first number 

2. the operation sign (addition or subtraction) point your feet towards the side of the the sign

      Addition = positive side of number line 

      Subtraction = negative side of number line 

3. if the number is positive walk forward or if the number is negative walk back wards

4. when done counting, put you feet at neutral 

An example:  

Start at 5 with neutral feet, point feet towards the negative side (subtraction), walk backwards 7 steps (-7), land on 12 with neutral feet. 

5-(-7)=12

One variation:

You could have one student move around the number line with an equation in their head and have the other students guess the equation that the student on the number line moved. This will show the connection between the operation signs and the number line. Next, a group students could come up with an equation and/or story and then the rest of their classmates have  act out the equation/story on the number line. 

Second variation:

In my college class, we played a game on the number line with a deck of cards. Take a deck of 52 cards and take out the face cards (Jack, King, Queen). A red card symbolizes a negative number and a black card represents a positive number. Pass out the remaining cards to each player, and have each player have the cards facing down. To start the game, each student will stand at zero and flip up their first card, the student with the smallest number will choose what side of the number line they wish to reach the end (negative side or positive side). The other student will be given the other side of the number line. The students then can give (subtraction) their number to the other student or move (addition) their number. This will continue until one student reaches the other end of the number line and win the game! 

Example: 

Student 1 and student 2 are starting at zero. Student flips up a -3 and student 2 flips up a 7. Student 1 has the smallest number between the two students and chooses to reach the end of the positive side. Then student 2 will have to reach the end of the negative side. The next flip, student 1 flips up a 4 and student 2 flips up a -9. Student 2 (going towards the negative) says he/she will "add -9" to himself/herself (0+-9=-9). While student 1 (going towards positive side) will "add +4" to himself/herself (0+4=4). Next turn, student 1 flips up a -6 and student 2 flips up a -4. Student 1 (going towards positive side) and will  "subtract -6" from student 2. Now student 2 will have to move -9-(-6)=-3. Now student 2 will "add -4" to himself/herself (-3+-4=-7). This continued until Student 2 won!